Is circle a polygon?

Jawad1 · December 25, 2021, 5:37pm

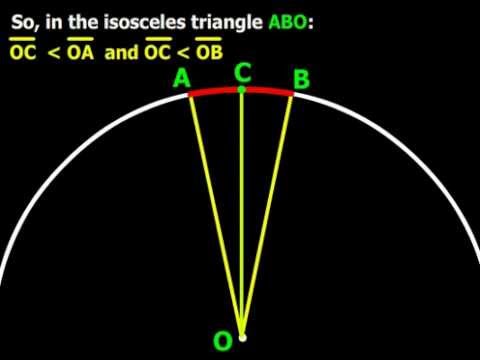
Is a circle a polygon? A polygon is a figure on the plane that is defined by a closed chain or circuit composed of a finite number of straight-line segments that are all the same length and close in on themselves. As a result, a circle cannot be classified as an equilateral polygon because it has no sides. As previously stated, a circle does not qualify as a polygon because it cannot be formed entirely from line segments.

Justification

The concept of concentric circles is another simple one to bring to your attention. Circular shapes with a single point in the middle are concentric circles. A circle is said to be concentric if it has the same center as another circle. Concentric circles can be formed if the circles have the same center.

Except for the circle, the “set” of points that are equidistant from a given location in two dimensions is referred to as a two-dimensional circle. A ring is an asymmetrically exploding point that has been reformed into a circle.

Others contend that the process has no corners because a corner requires generating at least three topics. Contrary to famous belief, a polygon is distinct from a circle.

A circle is a set of points on a plane equally spaced apart. The distance between the issue and the radius is called the radius of rotation. If you have a fixed number of the end-to-end line segments, you have a polygon.

It’s impossible to construct a circle out of line segments. Hence it doesn’t qualify as a polygon. This type of inquiry is easier to understand if you visualize the figure’s contours. The user determines the number of vertices in a polygon. A polygon can only be created if it has three sides (a triangle). There is no such thing as a side in a circle.

Gradually increasing the number of sides on a regular Polygon is an effective method for forming mental images of circles. Trigons are transformed from squares to triangles to yards to triangles to courts to pentagons to hexagons to heptagons and so on till they become squares.

Inscribe Or Circumscribe

Many geometric figures are created when circles are coupled with other geometric figures such as polygons. A polygon can be connected to a process in two ways. A circumscription is not the same as an inscription. Each of the vertices of a polygon inscribed in a circle is at right angles to the ring. Polygons surrounded by a process have all of their sides tangent to it.

Summary

Finally, I believe that mathematicians are responsible for examining the world around them. Math is merely a means to an end. It is simply a means to a lot like computers and even words. If a polygon with ever-increasing sides does not approach an actual circle, then it is an exaggeration. Circles are polygons with infinitely long sides. Our efforts were fruitful. The term “round” can be used to describe a point.

Polygon

Two-dimensional geometric shape with a finite number of sides. Several straight lines form the sides of a polygon. A polygon’s line segments are referred to as sides or edges because they are all connected. In geometry, a vertex (corner) is a point where two lines meet, and thus creates an angle. A triangle with three sides is an example of a polygon.

Even though a circle lies on a flat surface, it is not a polygon because it has no sides or angles. As a result, not all two-dimensional figures are polygons, even though polygons are all two-dimensional shapes. When it comes to polygonal shapes, we may use them consciously or unintentionally.

Shaped like A Polygon

Polygons are defined as a collection of lines. Several polygons are depicted in the accompanying diagram with varying quantities of line segments surrounding.

Different Polygon Shapes

Polygons can be categorized according to the number of sides and angles they have, such as:

Polygon with an Unusual Shape
A polygon with convex surfaces
Polygons with concave surfaces
Polygons with the same shape and size
polygon that is symmetrical in shape
A regular polygon’s sides and interior angles are all equal. Squares, rhomboid,
equilateral triangles and other regular polygons are examples of this shape type.

Polygons With Irregularities

Each side and angle of an irregular polygon are measured separately. There are numerous examples, including the scalene triangle and a kite.

Convex Surface.

If an angle in a convex polygon is smaller than 180 degrees, it is a convex one. The shape’s apex will point away from the center.

Concave Surfaces

It is a concave polygon if one or more of its interior angles exceeds 180 degrees. A polygon must have at least four hollow sides—the vertex points toward the Polygon’s center.
In contrast, the number of sides, angles, and properties of a polygon determine its number.

All The Sides Of Polygons

There is the same number of vertices and sides in any polygon. The angles in each corner are unique. The two polygonal curves are the interior and the exterior.

Interior Perspectives On Real Estate

A simple n-gon has (n 2) 180° in the sum of its internal angles.
In this case, (n 2) is equivalent to (n + 1)
Each Polygon has an equal number of sides, denoted by n.
The sum of all internal angles in a quadrilateral is:
The sum of the inner angles of a four-sided polygon is equal to (4 – 2) 180°
multiplied by two
It’s a full 360 degrees around.

Exterior Perspectives

Property with a view of the surrounding area
The sum of the interior and exterior angles at each vertex adds up to the total in each Polygon. In the example of a polygon;
There are a total of 180 degrees in a triangle when the inside and outside angles are added together.
When comparing interior and exterior angles, the difference is 180 degrees.

Characteristics

The properties of polygons are determined by their sides and angles.

• The sum of the internal angles in a polygon with n sides equals 180°.

• In a polygon with n sides, the number of diagonals is n(n – 3)/2.

• If you link the Polygon’s diagonals from one corner to the other, the number of triangles you get is n – 2.

• If you have n sides, the internal angle of the Polygon is [(n – 2) 180°]/n.

• The outer angles of an n-sided regular polygon are measured in 360°/n.

Summary

Polygons are two-dimensional geometric shapes with a finite number of sides and angles. In the following diagram, several polygons are depicted with varying quantities line segments surrounding them. Polygons have the same number of vertices and sides as they do angles. Its sides and angles determine the properties of a polygon. A simple n-gon has (n 2) 180° in the sum of its internal angles.

Formulas For Area And Perimeter

The length and width of a polygon’s sides are used to calculate its surface area, and Circumferencemferenceea of a polygon in a two-dimensional plane is defined as the area of the Polygon’s perimeter.

A polygon’s perimeter is the distance covered by all of its vertices.
The formulas for calculating the area and perimeter of polygons are as follows:

Polygon’s type	Formula of Area	Formula of Perimeter
Triangle	½ x (base) x (height)	Sum of all three sides
Square	side2	Sum of all four sides
Rectangle	Length x Breadth	2 multiply (length plus breadth)
Parallelogram	Base x Height	2 multiply (Sum of pair of adjacent sides)
Trapezoid	Area = 1/2 (sum of parallel side)height	Sum of all sides
Rhombus	½ (Product of diagonals)	4 x sum of all sides
Pentagon	14√5(5+2√5)side2145(5+25)side2	Sum of all five sides
Hexagon	3√3/2 (side)2	Sum of all six sides

Circle

A circle is a particular type of ellipse with zero eccentricity and two foci that coincide in mathematics or geometry. The Circumferencemference is the intersection of equal distances between two places.

The radii of a circle are the length from its center to its Circumferencemference the radius of a circle is the diameter, which divides the process into equal halves.

A circle is a simple two-dimensional shape that can be quantified in terms of its radius. Inside and outside sections of the plane are separated by rings. The line segment type is similar to this one. This example assumes that the line segment has been bent around to meet the two ends. By organizing the loop in a circle, you can circular it.

There are two dimensions to a circle: it’s diameter and its area. The distance encircling a ring is its [Circumferencemference//howtodiscuss.com/t/circumference-of-cylinder/79050) or perimeter. In a two-dimensional plane, the size of a process is in the place it encompasses.

In the plane, the circular formula is:

(x-h) 2 + (x-h) 2 (y-k)
The value of r2 is equal to two.
The coordinates (x,y) are the x and y coordinates.
The center of a circle’s coordinates is (h,k).
The letter r indicates the radius of a circle.

Objects Shaped Like A Circle

We’ve seen a lot of circular items in the actual world. Here are a few examples of this:

• A ring

• Media Type: Compact Disk/Digital Video

• Necklaces and bracelets

• Currency

• Tires

• Toggle switch

• Darts with a dartboard

• Hula hoops are used for dancing and are made of plastic or metal.

Circle Characteristics

The following are some of the most fundamental properties of circles:

• Every point on the circle’s Circumferencemferencel in distance from the center.

• The circle’s diameter divides it into two equal pieces.

• Congruent circles have the same radius.

• In terms of radii, all circular shapes have the same characteristics.

• The circle’s diameter is double the radius; hence the diameter is the most significant chord.

To Draw A Circle

Math projects, such as building a circle, clearly explain the qualities and terminologies. To make sense of processes in a non-technical way,

• On a blank piece of paper, draw a single point and mark it Point O.

• For the radius, choose a random length, such as 3 cm.

• Measure three centimeters away from point O, keeping the zero mark at point O.

• Keep the distance from point O to a maximum of three centimeters while making your markings.

• To get a circle-like shape, you’ll need to select many points.

Circle’s Sub-Components

Several distinct sections in a circle are based on their location and characteristics. A process is made up of a variety of different parts.

• Two concentric circles are used to define the annulus. To put it another way, it’s a ring.

• Circles are made up of equal and opposite sides.

• This type of section is defined by two radii that are joined by an arc, as well as radii that are joined by an angle.

• An arc that connects the ends of a chord is called a segment. It’s worth noting that chunks don’t have a central reference point.

• In a circle, the midway point is the center.

• It is a chord if the ends of the line segment are on a circle.

• A circle’s diameter is a line segment that connects the circle’s two endpoints and is the most significant chord of the process.

• A line segment that extends from a circle’s center to any other point on the process is known as a radius.

• A straight line divides a circle into two equal parts: An extended chord is another term for it.

• A tangent is a direct line that touches a circle just once.

• The radius of CircumferencemferenceTo represent the circle’s radius, we use the letters “R” or “r,” which stand for “radius” in math.

A Circle’s Diameter (d)

Both of the endpoints of a line segment are on the circle. D is equal to 2r, where r is the diameter of the process. The formula r= d/2, derived from the diameter, yields the circle’s radius.

Formulas In Circles

Using two different parameters, a circle’s two-dimensional curve shape can be quantified:

The surface of a ring
The diameter of a circle’s Circumferencemferenceummary
A circle is a particular type of ellipse with zero eccentricity and two foci that coincide in mathematics. A circle has two dimensions: its diameter and area.
In a two-dimensional plane, the size of a process is in the place it encompasses. Circles are made up of equal and opposite sides.
A line segment that extends from a circle’s center to any other point on the process is known as a radius. The formula r= d/2, which is derived from the diameter, yields the circle’s radius.

A circle’s Circumference And Area

Circumference (C)
• Measurement of the Circumference (C)

• It is equal to the Circumferencemferencercle plus the Circumferencemferencecircle plus the
Circumference of the process plus the Circumferencemferencercle.

• A ‘perimeter’ is a term used to describe a line that connects two points.

• However, it is not as common as the other options.

• When it comes to polygons, the distance surrounding them is referred to as

• Calculating the circle’s Circumference can be done using the following formula.

There are two ways of looking at this equation

You can look at it as the sum of two numbers.
You can look at it as the sum of two integers.
You can look at it as the sum of two natural numbers.

C = πd = 2 π r
Where, π = 3.1415
Area (A)
The volume of space occupied by a circle defines its area (A).
Using this formula, the area of a circle is calculated.
Area of a circle = πr2

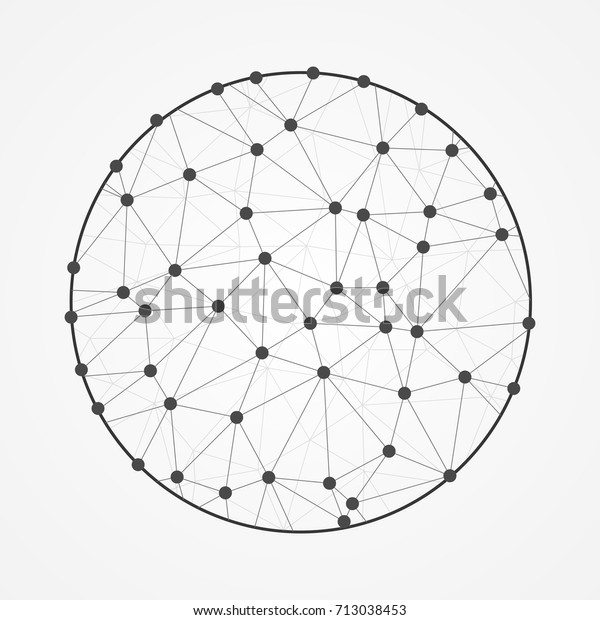
The Circle Area Can Be Seen Here.

• A circle’s area is the amount of space it occupies.

• Consider a concentric circle with a radius of r.

• Make a right-angled triangle by opening all of the circles in the concentric circle.

• The base would consist of an outer circle and a line of length 2r.

• ‘r’ would be the suffix for the number of characters.

• Consequently, a circle has the same area as a right-angled triangle.

• The following are the results: (1/2) bh

• It is equivalent to a half (half) = 2 r (half) = 2 r (half) = 2 *22/7 r r (half)

• Consequently, the circle’s area equals =22/7r^2.

Frequently Asked Questions (FAQ)

People asked these questions frequently

1. Is a circle the only shape that isn’t polygonal?

Straight lines on a flat surface, such as paper, can create a polygon. Squares, rectangles, triangles, and pentagons are examples of these shapes, but circles and other curved shapes are not.

2. Besides polygons, what other shapes exist?

Perpendicularity refers to the angle formed by the intersection of two parallel lines. It is up to the user to choose the number of vertices a polygon has. A polygon is a contained object if its sides are all straight.

3. What does it mean when you say a circle?

There are no corners or edges to a circle, and all locations on its surface are equal distances away from its center.

4. What are the various portions of a circle like in terms of appearance?

The radius, diameter, chord, tangent, arc, center, secant, and circle sectors are separate.

5. Take note of the circle formulas that you need to write down.

If “r” is the radius of a circle, then:
The diameter of a circle is equal to 2r units.
The area of a circle is equal to r2 square units.

6. The radius and diameter of a circle must be defined and their length.

It is the line from where the center point is to where the circle is on the surface. There are two things you need to know about the diameter of a circle.

7. What is a chord?

Straight lines with both ends touching the surface of a circle make up the chord of a circle. There is a diameter for every long chord in a circle.
Distance and the point are passing through the center.

8. Describe Polygon?

A polygon is a shape that has a lot of different sides.
A polygon is a closed two-dimensional shape that is made by putting lines together. A polygon must have at least three lines to make it.

9. A polygon with seven sides defines it?

A polygon with seven sides is called that because it has many sides together.
You can think of it as a Heptagon, which means it has seven sides. This Polygon has seven sides.

10. A polygon with nine sides defines it?

A polygon with nine sides is called that because it has many sides.
As you can see, it has nine sides. This is a Nonagon. This Polygon has nine sides.

11. How many diagonals does the Polygon have?

This means that for every side in the Polygon, there must be an equal number of diagonals. This is called the number of diagonals divided by three. In triangles, there are no diagonals, so there are no triangles

12. What are the various sorts of polygons?

Polygons are categorized mostly into four types. They are as follows:
Regular Polygon - all sides and interior angle measurements are equal.
Irregular polygon - all sides and interior angle measurements are not identical, i.e., different
Convex Polygon - a polygon whose internal angles are all smaller than 180 degrees.** The vertex will radiate from the shape’s center.
Concave Polygon — a polygon with one or more interior angles greater than 180 degrees. A concave polygon can have a minimum of four sides. The vertex is pointing inside the Polygon.

Conclusion

Circular shapes with a single point in the middle are concentric circles. A circle is said to be concentric if it has the same center as another circle. A polygon is a closed chain or circuit consisting of a finite number of straight-line segments.
A polygon can be connected to a process in two ways. Polygons surrounded by a process have all of their sides’ tangent to it. Circles are polygons with infinitely long sides - there is no such thing as a “side” in a circle.