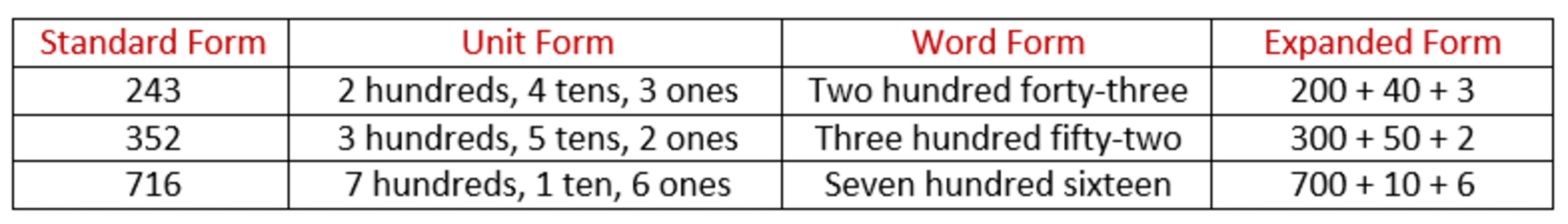
Unit Form

Rabail.waqar · October 15, 2022, 7:38am

Unit Form

Unit form is an alternative to standard form of mathematical equations, which looks like y = mx + b, where ‘m’ is the slope and ‘b’ is the y-intercept (the x-value where the line crosses the y-axis).

The Unit Form

In mathematics, unit form is a way of writing numbers to reflect the number of units that make up the whole. For example, in base 10, the number three hundred ninety-seven would be written as 3’397. The 3 is simply a placeholder for thirty, the 9 is a placeholder for nine, and so on. In this example, there are four units that make up the whole (thirties, nines, tens and ones).

Types Of Unit Form:

There are three types of unit form in mathematics. The first is the most common, and it uses the number one. This type is called improper unit form. The second type of unit form is called proper unit form and uses the number one to represent a measurement with an unlimited number of digits after the decimal point. The third type is called mixed unit form, which also uses the number one, but as a stand-in for a digit or digits that have not yet been chosen to represent a certain measurement.

Conversions in Mathematics:

There are a number of different types of conversions in mathematics. These include: 1) unit form; 2) number form; 3) power form, and 4) decimal form. Unit conversion is the process by which you convert from one unit to another, like from feet to meters. Number conversion is the process by which you convert from one numeric system to another, such as converting from base-10 to binary. Power conversion is the process by which you transform a number raised to a power into its equivalent value not raised to a power (e.g., 64 = 8), and decimal conversion is the process by which you transform an integer into a decimal without rounding off any digits past the decimal point (e.g., 342 = 342.00).

Unit Conversions:

In order to make units conversions more useful, a system of unit form has been created. The system is based on the dimensions of length, weight and volume. Units of different lengths are expressed in meters, units of different weights are expressed in kilograms, and units of different volumes are expressed in liters.
In order to convert from one type of unit to another, you need to know what number is being multiplied by the conversion factor. For example, if someone weighs 200 pounds and wants to convert that into kilograms, they will multiply 200 by 2.2 because there are 2.2 pounds per kilogram.

How to Convert Fractions into Decimals

To convert a fraction into a decimal, divide the numerator (top number) by the denominator (bottom number). The result will be your decimal. For example, if you wanted to convert 3⁄4 into a decimal, divide 3 by 4 to get 0.75.
If you wanted to convert 9/10 into a decimal, divide 9 by 10 to get 0.9. Converting fractions can also be done with a calculator. However, it is often easier to do it mentally first before converting it. For example, if you had 1⁄5 and wanted to know how many decimals there were in this fraction without using your calculator: divide 5 by 5 to get 1 and that would then tell you that 1 of these fractions equals one tenth and there are five in all.

How to Convert Decimals into Fractions

To convert a decimal to a fraction, first convert the decimal to a fraction by finding its unit form. To do this, multiply the decimal’s digits by their respective powers of 10. For example, to find the unit form for 0.5: 0.5 = 5/10 (or 1/2). To find the unit form for 0.6: 0.6 = 6/10 (or 2/3). There are four basic steps in order to find the unit form of any decimal number. Step One is to express your decimal number as a fraction with denominator 10 and numerator 100 (For example, .625= 6/100). Next, subtract from that total all non-zero digits after the decimal point and add these new numbers together. Then reduce if necessary and lastly simplify your final answer if possible.

How do you convert a fraction into a percentage?

First, find the decimal equivalent of the fraction. To convert a fraction to a percentage, simply multiply it by 100% and add a % sign to the end. For example:
1/2 x 100% = 50% (since 1/2 x 100% is 50)
3/4 x 100% = 75% (since 3/4 x 100% is 75)
1/8 x 100% = 12.5% (since 1/8 x 100%) is 12.5)
To convert decimals into percentages, take the decimal equivalent and divide it by 10%. You will then have your percentage.

How do you convert a decimal into a percentage?

To convert a decimal into a percentage, there are two ways to go about it. First, you can multiply the decimal by 100 and then add the desired percentage sign. The other way is to divide the decimal by 100 and then add the desired percentage sign. For example, if we wanted to convert .3 into a percentage, we would first multiply by 100 (.3 * 100) which equals 30 and then add the percent symbol (30%). Alternatively, if we wanted to convert .3 into a percentage, we would divide by 100 (.3 / 100) which equals 3% and then add the percent symbol (3%).

Understanding unit form in mathematics

The unit form of a number is simply the quotient (a fraction) obtained by dividing that number by 1. For example, in the unit form of 5, we have 5/1 = 5. In the unit form of 3 we have 3/1 = 3. The unit form of a number can be used to express fractions and mixed numbers as well. For instance, in the unit form of 6 and 7, we would write 6/7 or 2/3 as it relates to decimal equivalents. Unit forms are also useful when working with percent, for example 50% would be written as .5 (or 50 ÷ 100). They can also represent exponents of base ten; if you want to raise 3 to the power 4, you will use 32 (which equals 27 in base 10). And finally, they are helpful when dealing with large values.

Converting from Quotient form to unit form (or vice versa)

Quotient form converts to unit form by finding the common denominator for both numbers and then dividing them. For instance, say we wanted to convert 12/3=4 into unit form. We would take the numerator (1) and the denominator (2) and divide them. The quotient is 1, which we round up to 2 in unit form because of the rule that an answer should be as close as possible to a whole number without going over.
The reverse process can be done with division, so if we wanted to find the quotient of 4/2, we would take two steps: first divide 4 by 2, and then divide that answer by 3. This gives us a quotient of 1 with a remainder of 1 in unit form.

What is Unit Form?

In mathematics, unit form is the representation of a number in a simple one-to-one correspondence with an element of the set of real numbers. For example, formula_1 and formula_2 are unit forms. Unit form can be thought to correspond to the value that’s measured when calculating area or volume (e.g., lengths), but these values are usually not written in unit form because they’re usually understood without having to write them down (i.e., 1 meter).

Uses of Unit Form in Mathematics

In the study of mathematics, unit form is a particular representation of an object by displaying it as a number multiplied by one. It is also used for functions that have only one input and one output. Unit form can be used in solving equations as well as simplifying fractions. It is especially helpful in simplifying fractions with denominators consisting of repeating decimals. For example, 3/8 simplified to 1/2. You can use unit form to find solutions to equations as well because they are linear in nature. For instance, if you had 5x=3, then x=2 and 5x=6. The use of unit form is often limited to counting numbers like 2 or 7 and not numbers like -5 or 13/4 because negative numbers cannot be made positive using this method.

What is Decimal Unit Form?

Decimal unit form is a type of unit that uses decimals instead of fractions. Decimals are a number between 0 and 1, while fractions can be any other number. To convert from fraction to decimal, divide the fraction by the denominator. For example, if you want to convert 2/5ths to decimal form: 2 divided by 5 = .4 (or four tenths)
Continuation (six+ sentences): A whole number with a decimal point after it represents how many tenths there are in the whole; in this case, four. Any number less than one would represent hundredths or thousandths.
Continuation (six+ sentences): Numbers after the decimal point show what part of the whole we’re talking about, so in our example above two tenths would be written as .2 because two goes into 10 three times with a remainder of two. In our examples above we were only talking about integer numbers- whole numbers without fractions- but this process works for any real numbers- numbers which include fractions and decimals

How Do You Write 170 in Unit Form?

170 can be written in unit form as 1.7 × 10^2 = 17. There are many different ways to write a number in unit form, and students learn how to do this in elementary school math. Unit form is often used for scientific notation, which is a way of writing really big or really small numbers so that they are easier to understand. The exponent on the unit refers to how many times 10 needs to be multiplied by itself in order for the number being expressed to equal that number in decimal form. In this case, 170 would need to be multiplied by itself 17 times before it equals 1.7 × 10^2 = 170

How Do You Write 40 in Unit Form?

In order to write 40 in unit form, you need to convert the number from decimal form to unit form. To do this, you will divide the number by the appropriate conversion factor. For example, if you are trying to convert 40 into units of meters, then divide 40 by 1 meter because there is 1 meter in every unit. The answer will be 4 meters.

Examples of Unit Forms:

There are two types of Unit Forms: Unit symbols and Unit names. One example of a unit symbol is the letter c. A person can also use a word to represent units, such as hour or kilometer. Examples of unit names are Celsius and Fahrenheit. When we use words to represent units, it is important that we spell them correctly and capitalize them, so they stand out from other words in the sentence.

The Most Widely Used Unit Form:

The most widely used unit form is the SI, or International System of Units. It is a decimal-based system of units that defines seven base units: the kilogram, gram, mole, ampere, kelvin, candela and mole. The SI was created in 1960 at the 11th General Conference on Weights and Measures (CGPM). Originally called Le Système international dunnites (French for The International System of Units), it abbreviates to SI from French and to Système international from English.

The SI:

The International System of Units (SI) is a system of units that was created to provide a consistent, coherent and rationalized system of measurements. It uses seven base units: the meter for length, the kilogram for mass, the second for time, the ampere for electric current, the kelvin for temperature, and the candela as a unit of luminous intensity. The name SI comes from its French name le Système international d’unités where international refers to its status as an international standard

Conversions in SI:

Units of measurement are necessary when dealing with the physical sciences. All measurements must be in the same units for any given problem. In order to convert from one type of unit to another, you can use a number conversion chart. This is an easy way to see what equals what in terms of units. You will need to know the specific unit you want to convert and also the measurement that you want it converted into.
For example, say we have a rock that weighs 1 kilogram and wants to know how many pounds it is worth: (1 kg = 2.2 lb). Simply find the kilogram column and follow it across until you get to 2.2 pounds, which would be 44 pounds in this case (1 kg = 2 lb.).

Summary:

In math, the unit form is an expression in which a number is multiplied by one. This kind of expression can be written as a fraction in which the numerator and denominator are both equal to one. Unit form is also referred to as unit fractions, because multiplying by one makes any number into a fraction with denominator 1. When you multiply any number (other than 0) by 1, you will always get that same number back. Examples of unit form include 4/1, 5/1, 8/1, and so on.

Frequently Asked Questions (FAQs):

1. What is the unit form?

The unit form of a number is its numerical representation as a single digit. For example, the number nine can be written as 9 in decimal form or 2 in binary form. However, when you write it in this way, it doesn’t really help with your understanding of the unit part of unit form. When you write numbers using only one digit, such as 1 or 4, that’s what’s called the unit part of the number because it’s just one digit and not a string of digits like 45. If you have to convert an integer to a floating-point number for some reason (like dividing two integers), then you will need to use the unit form.

2. What are some examples of using a unit form?

If you were asked to divide 7 by 3, then you’d need to use a unit part of some sort because 3 is not made up of one digit. In this case, you could write it as 1/3 or .33333… You’ll also see people adding exponents to a number in order to make it smaller or larger. For example, one billion can be written as 1E9 and two hundred thousand can be written as 2E6. In these cases, E is used as an exponent (with E standing for times 10), so we have 10 raised to a power of nine and 10 raised to a power of six respectively.

3. Why is unit form important?

Using a unit form in your calculations can be very useful because it lets you add and subtract without having to worry about how many decimal places are in your number. For example, if you were told to find out what seven hundred and three is in scientific notation, then you could simply multiply 7 by 10 raised to a power of three (or 7 × 103), which would give you 7 × 103 = 7000. If there was no exponent on that number, then it would be more difficult to work out since it’s not as obvious that 3 divided by 10 gives 0.3 and multiplying that result by 10 gives 3.

4. How can I calculate a unit form?

To find the unit form of a number, you can follow these steps:
Find all possible factors of the number except for itself. All those factors combined should equal to one less than the original number minus one.
Multiply each factor by ten and add them together.
Take away any zeros that may appear at the end of your answer and keep adding on ‘1’.

5. How can I use unit form in my calculations?

When working with unit form, the numbers on either side of the equation must contain the same number of digits. Unit form makes addition easier and helps you avoid carrying decimals into subsequent calculations. When solving equations with multiplication or division problems in them, remember that you can’t mix units when doing calculations. Be sure to convert everything into the same units before beginning your calculation.

6. How do I convert between unit forms?

If you want to convert from decimal form to binary form, the procedure is similar to converting from decimal form to hexadecimal. Divide the number by two and drop any remainder. Repeat until zero appears in the quotient. Note that you should drop all non-zero digits of the dividend during this process.

7. How do I convert from hexadecimal form to binary form?

First divide the number by sixteen and drop any remainders. Drop all non-zero digits in the dividend during this process. Next, repeat this step until you have a zero in your quotient. Then round off your final number up to nearest whole digit. Finally, remove any trailing zeros from the answer.

8. How do I convert hex to decimal?

First find out how many digits are in your hex number. To do that, count how many powers of sixteen are multiplied by a power of two (one with an exponent of 16 and one without) in your answer. If you only see one, then it’s five digits long. Add one more if there are three entries or two more if there are four entries - add all together to get your total digit count.

9. How can I calculate binary to decimal?

The first step is to determine how many digits are in the number. Count the powers of two (with exponents) times a power of sixteen that have been multiplied together. If there are only one or two, then it will have five digits; but if there are three or four, then it will have six digits. Keep in mind that you need to take away 1 from your answer when counting.
To find out how many digits make up binary, just put 0s wherever 1s were previously located and start over again at 9 so that you know where to stop counting. The difference between binary and decimal is after getting back down to 5 through 9, we start counting 101 instead of 10.

10. What is 50 in Unit Form?

The easiest way to find the answer is to break it down as follows:
Find all possible factors of fifty which includes 2, 4, 5, 10 and 25. That equals 100 which would be too much because fifty needs to be divided by 1 less than itself (-1). The next best option would be 3 since 3*10=30+5=35+5=40.

Conclusion:

In math, unit form is where a number is multiplied by 1. For example, 3 x 1 = 3. Unit form can be used for any numbers that are not zero. Unit form can be used to simplify an expression and make it more manageable or easier to solve or do arithmetic with.