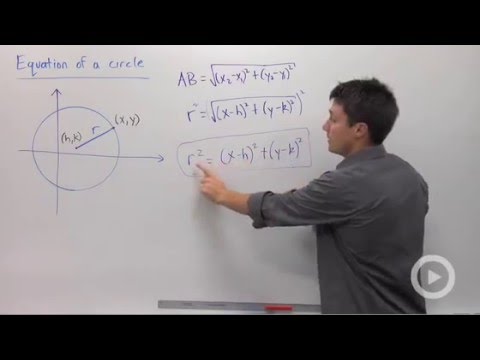
Equation Of a Circle. There are three different forms of the equation of a circle that can be used to define its radius and centre or point of origin. Depending on the form you need, you can use either the distance formula or the midpoint formula to find the coordinates of the centre of your circle. The radius, r, can then be found with either the distance formula or by taking half of the length between (x1, y1) and (x2, y2).
EQUATION OF A CIRCLE
Every circle has a radius, so r is the length from the centre to any point on the edge. The x-axis is drawn perpendicular to the radius and intersects with it at two points. One issue is where y=0 (the centre), and one end is where y=r (the edge).
The equation for a circle centred at (h,k) with radius r can be written as: (x-h)2+(y-k)2 = r2. This implies that all points in a circle are equidistant from the centre. The distance between the point’s location and the centre can be found by subtracting h or k from r.
When h=k, then x2+y2 = r2 becomes (x-h)2+(y-k)2 = 0 or (-h+k) = 0 or h=k. So all points on a circle centred at (h, k) are either on its edge or centre.
In general, given any point (x, y) on a circle of radius r and centre (h, k), it’s equation is x2+y2 = r2 - h2 + k2. The distance from that point to (h,k) can be found by subtracting h or k from r.
If we let C be any point on a circle with radius r, (h1, k1) and (h2, k2) are two different points on that circle. If h1 = h2 and k1 = k2, then C is on that circle. For example, let’s use (9,-12),(-3,-6),(-7,-8) to represent three distinct points which satisfy x2+y2=9.
EQUATION OF A CIRCLE WITH CENTER AND RADIUS
A circle is defined by its centre point and radius. The equation for a process is r = √(x2 + y2), where x and y are the coordinates on the plane. For example, if the coordinate (x,y) lies in quadrant I, then
on the unit circle with a radius one centred at the origin, x=1, y=-1/√3=-√3, and we have r=√(-√3-1)=1
The equation of a circle is x2+ y2 = r2, and The general equation for a loop with a centre point (x0, y0) and radius r is (x- x0)2 + (y- y0)2 = r2. Here, we want to find out how to determine if an arbitrary point(x,y) on a plane lies inside or outside a circle.
We define R_min and R_max as follows : Then, if x- x0 2 + y- y0 2 > R_max, then (x,y) lies outside our circle. Similarly, if x- x0 2 + y- y0 2 < R_min, then (x,y) lies inside our circle.
Now, we can re-write our earlier equation as x - x0 2 + y - y0 2 = (R_max - r2)/(2R_min) and compare it with the cosecant function to find out if (x,y) lies inside or outside the circle.
If x0 is not equal to 0, then the cosecant function will have values less than 0 for (x - x0)/(x0), meaning our point lies outside the circle.
Similarly, if y0 is not equal to 0, then the cosecant function will have values greater than 0 for (y - y0)/(y0), meaning our point lies inside the circle.
EQUATION OF A CIRCLE GIVEN TWO POINTS
The equation of the circle with centre at (h,k) and radius r is given by the following formula:
x^2 + y^2 = r^2 . Let’s explore some examples. Knowing the coordinates for two points on the plane can determine what kind of curve they form.
In this example, let’s say that one point has coordinates (-5,-5), and another has coordinates (4,-4). We can use these points to create a circle with a centre at (-5,-5) and a radius of 2.
Linear equations connect the coordinates for points on a circle. Let’s say that we have three points that make up our curve, each with its own distinct set of x and y coordinates. We know that these are points on a circle when they fall within an x-y coordinate plane.
The equation to describe such a circle is given by x^2 + y^2 - 4x - 4y + 16 = 0. If you simplify the equation using the quadratic formula, it becomes 2(x+1)(x-1) - 4(x+1)(y+1)(x+1) -4(x+1)(y-1)(x-1)=0.
We can solve for (x-1) and (y+1), so that we have: x^2 + y^2 - 4x - 4y + 16 = 0. Now, let’s put our values in (-1)^2 + y^2 - 4(-1) - 4(4) + 16 = 0. We now know that our circle has the equation: (-1)-4•4+(16)=0.
Let’s start by using points (1,1) and (-2,-2). We know that: 1^2 + (-2)^2 - 4(1)(-2) - 4(-2)(1) + 16 = 0. With these numbers plugged in, we learn that our equation is: 1-4•-2+16=0. Next, if we take (3,7) and (-8,-8), then the result will be 3^2 + (-8)^2 - 4(3)(-8) - 4(-8)(3) + 16 = 0.
EQUATION OF A CIRCLE DESMOS
When discussing the equation for a circle, we assume that the centre is the point (h, k) with coordinates (h, 0) and that the radius is r.
We also assume that our circle has no vertical or horizontal tangents. Any line drawn through the centre will intersect with only one point on each side.
For an ellipse, we do not assume that there are no tangents, which means that any line drawn through either centre will intersect with at least one point on each side. This means that our formula for r is a function of both t and φ
You can see from the figure that formula_5 is parallel to formula_6 and perpendicular to formula_7.
This means that because they are perpendicular to each other, we can use properties of right triangles to solve for our unknowns.
Because both sides of our triangle are radii, we know they are congruent by SSS (Side-Side-Side) Postulate. We see that side adjacent = adjacent over hypotenuse and opposite = opposite over hypotenuse.
We know that because both sides are radii, we can use Pythagorean Theorem to solve for formula_10 and formula_11, which means that we now have enough information to write our final equation. From here, we can find r, t, and φ.
EQUATION OF A CIRCLE EXPLAINED
The equation for a circle is (x-h)2+(y-k)2=r2. It has a centre point (h,k) and a radius, r. This formula can be used to find the equation for any point on the circle by plugging in the coordinates for that point into the recipe.
In contrast, (x-h)2+(y-k)2 is just equal to (x-h+r)2+(y-k+r)2; r is cancelled out because each side has it and thus simplifies to x2 + y 2 = r 2. Now we can put in any values for x and y that we please and get our circle.
We take square roots on both sides to get our centre point, h and k. This will give us (h+r/2) and (k+r/2). We then add r / 2 to each side to ensure that our circle is centred at our coordinate.
We can use our equation to create any point on our circle by plugging in coordinates. A good example is (3,5).
Plugging those into our equation gives us: 32 + 52 = 25 + 25 = 50. Thus, (3,5) is an equation for our circle. To put that another way, 3 and 5 are X and Y coordinates for a point in our process, and 50 is its radius.
HOW TO FIND THE EQUATION OF A CIRCLE
To find the equation of a circle, use the radius and any point on the process as your coordinates. Here’s how it works for a loop with centre (h, k) and radius r: x^2 + y^2 = h^2 + k^2 - 2rhk + r^2 FINDING THE EQUATION OF A CIRCLE WITHOUT CENTER POINT:
If you don’t have an intersection point or if the intersection is outside of the circle, we can use (0, 0). In this case, you would still need to know the radius to calculate other information, such as circumference or area. Here’s what that looks like x^2 + y^2 = 0
If you already have an intersection point, that’s awesome! Here’s what it looks like: (x1, y1) ^2 + (x2, y2) ^2 = r^2. So using our same example where the equation of a circle with centre (h, k) and radius r is x^2 + y^2 = h^2 + k^2 - 2rhk + r^2, our values are replaced by x1= 4, y1= 8, h= 4, k=-6.
And our equation is 4^2 + 8^2 = 16 - 2(4)(-6) + 9^2 = 0. So the equation of our circle would be (x, y) = (-5, 5). That’s it! Now you know how to find an equation for any circle!
So how do you use that equation to find things like area or circumference? Let’s walk through it. First, we must replace x and y with our centre point (h, k). Here’s what that looks like: h^2 + k^2 - 2rhk + r^2 = (4)^2 + (-6)^2 - 2(4)(-6) + 9^2 = 36.
EQUATION OF A CIRCLE EXAMPLES WITH ANSWERS
The equation for the circle with centre at (h, k) and radius r is given by the following equation: x^2 + y^2 = r^2. For example, if we want to find the equation for a circle with a centre at (-1,-1) and radius 3, we would plug in h=-1 and k=-1.
Another way to view circle equations is as circles pass through (h, k). In other words, if h and k are real numbers, we can write an equation for every point on that circle.
By plugging in (-1,-1) into our original equation, we get x^2 + y^2 = r^2. This shows that all points along our circle satisfy the equation x^2 + y^2 = r^2.
Using x = (h - k)^2 and y = 2k, we get a circle that passes through (-1,-1). In other words, all points on our circle are given by x^2 + y^2 = (-1-0)^2+ 2*(-1)*0 = 1. So plugging in (0,-3), for example, we see that its coordinates satisfy our equation.
With any value we plug in for h and k, both x and y satisfy our equation, as do all coordinates. For example, if we plug (1,-2) into our original equation x^2 + y^2 = r^2 we find that (1,-2) satisfies both x^2+y^2=r^2 and 2(1)(-3) + (-12) = -13.
| In contrast, (x-h)2+(y-k)2 is just equal to (x-h+r)2+(y-k+r)2; | We take square roots on both sides to get our centre point, h and k. |
|---|---|
| Cancelled out because each side has it and thus simplifies to x2 + y 2 = r 2. | This will give us (h+r/2) and (k+r/2). We then add r / 2 to each side. |
| Now we can put in any values for x and y that we please and get our circle. | To ensure that our circle is centred at our coordinate. |
GENERAL EQUATION OF A CIRCLE CALCULATOR
We must first find the radius to find the equation for a circle. To do this, use the following formula:
r = √(x^2 + y^2) Once you’ve found the radius, plug it into one of these two equations: (x- h)^2+(y-k)^2=r^2 or (x-h)^2+ (y+k) ^ 2=r ^ 2.
(x-h)^2+(y-k)^2=r^2 or (x-h)^2+ (y+k) ^ 2=r ^ 2 If you aren’t sure which equation to use, plug in each set of variables and compare the results. If one of them works, you’ve got your equation for a circle!
The circle equation (x-h)^2+(y-k)^2=r^2 is often referred to as quadrantal, and (x-h)^2+ (y+k) ^ 2=r ^ 2 is referred to as polar.
Which one you use doesn’t really matter. Some equations are more accessible to memorize, but either way will get you a circle!
If you prefer using degrees, we have an alternative equation for you! You may be more familiar with (x-h)^2+(y-k)^2=(r * 2 * pi)^2 or (x-h)^2+(y-k)^2=(r * 2pi) ^ 2, and that’s totally fine. It’ll get you a circle no matter what! Just make sure to plug in radians for r and your variables.
EQUATION OF A CIRCLE GIVEN CENTER AND POINT
The equation for a circle with center (h, k) and point (x, y) on the circle is (x^2 + y^2 = h^2) The equation can also be written as:
(x^2 + y^2 = r^2) Where r is the radius. You can also find this relationship by looking at the distance formula to solve for x. First, we substitute (x, y) for each variable. (d = \sqrt{(x - h)^2 + (y - k)^2}) Now we square both sides.
(d^2 = \sqrt{(x - h)^2 + (y - k)^2}) Then take square root on both sides. (d = \sqrt{r^2}) Multiply both sides by r.
(r^2 = d) Solve for r. (r = \sqrt{d}) And that’s how to find x when given center and point. To use these methods, you must already know how to find the centre and radius in a circle.
The video tutorial below covers finding these values in detail. The methods are discussed in detail below as well.
Finding Center and Radius using Pythagorean Theorem The video below is a detailed step-by-step tutorial on how to find the centre and radius for any circle, given its centre and point.
If you don’t know how to do that, check out that video before attempting these methods.
Get to our equation for finding x when given the centre and point. As we saw in that video, there are two ways you can use the distance formula to solve for x.

Summary
The equation for a circle is (x-h)2+(y-k)2=r2 where x, y, and r are the coordinates. The centre (h,k) is found by solving for either x or y first. A circle can also represent an equation with the form (x+w)2+(y+h)2=r2.
Frequently Asked Questions
Some Important Questions For Your Knowledge:
1) How do we find the equation of a circle?
A circle is the set of points at a given distance from one point. The equation for the circle with centre (0,0) at the origin and radius r is x2 + y2 = r2. This can be rewritten as x2 + y2 - 2rx = 0. We can also write this as x2 + y2 = ±r2. These two equations represent the total possible solutions for this problem!
2) What is the equation of a circle GCSE?
The equation for a circle with center ((h, k)) and radius (r) is:$$x^2 + y^2 = r^2$$ $$\begin{aligned}&x = \sqrt{h^2+k^2-r^2} \&y = \sqrt{h^2+k^2-r^2}\end{aligned} $$ The two points on the circumference are given by (\left(\frac{\pi}{2}, 0\right)) and (\left(\frac{3\pi}{2}, 0\right)).
3) What are the three circle formulas?
The formula for the circumference is 2πr. The formula for the area is πr2. And the formula for the radius is r. A circle with an equation x2+y2=1 has both of these circles formulas because it can be graphed in two different ways: from x=0 to x=-1 and y=-1 to y=0 or from x=-1 to x=0 and y=-1 to y=0, depending on which one you want to use as the outer circle or inner circle.
4) What is the standard form of the equation of a circle?
The equation for the equation of a circle is: (x-h)2+(y-k)2=r^2. The graph shows the x, y, and r coordinates, and h and k are the x and y intercepts. It is important to note that this expression holds for any point on the chart with an x value greater than or equal to h and less than or equal to k.
5) How do we find the radius of a circle?
To see the radius of a process, we use the equation for the circumference of the process and divide it by 2π. The radius is halfway between the centre and any point on the edge. To find the centre, we draw two perpendicular lines from any point on the border to either side of our circle.
6) How do you find the equation of a circle given two points?
The equation for a circle is x2 + y2 = r2. To find the equation of a circle given two points, you need to solve for r in the following equation: x - (y/r) = 0 and y - (x/r) = 0. These equations can be solved using substitution and linear algebra. For example, if we have x = 3 and y = 4, then r would equal 9.
7) What is the general form of an equation?
The equation for the equation of a circle is as follows: (x-h)2+(y-k)2=r2. It is called the general form because it can be used to find any point on the circle, not just the centre. To find the distance between two points, use the following formula: d=sqrt((x1-x2)^2+(y1-y2)^2).
8) How do you form equations?
The equation for a circle with a centre is the following: X^2 + Y^2 = R^2 where R = distance from the centre to any point on the circle’s circumference. The radius (R) can be either positive or negative. WOW, that was easy! To get an equation in standard form, you only need to solve it and put it into parentheses! For example, let’s say we have 4x - 3y = 2, so what we do is multiplie diply by y, then add 3 to both sides, so we get 4x - 3y + 3y = 6y, which then equals y(4x-3).
9) What are the two equations of a line?
The equation for the circle’s centre is ‘x^2+y^2=r^2’ where ‘r’ is the radius, and the equation for a point on the circumference of the circle is (x-h)^2+(y-k)^2=(r+h)^2. If you know any two points on the process, you can find its radius theiry taking their difference squared.
10) What is an equation for a line?
An equation for a line is y = MX + b, where x and y are the coordinates of the line. The slope, or steepness, of the line is represented by m, and b is the y-intercept.
Conclusion
The equation for a circle is found by finding the centre, then plugging it into the radius and drawing a line from the centre to any point on the circumference. The radius of the circle is equal to its distance from the centre. A circle has an infinite number of points on its circumference, but only one point t called this e centre.